March 5, 2023February 3, 2024 by Beyond Traditional Math

Fun Ways To Teach Equivalent Fractions And Make It Stick For Good

If you have stumbled across this blog post looking for equivalent fractions worksheets, you may want to go back to your google search because you really won’t find worksheets here. What you will find is a packed post full of fun ways to teach an understanding of equivalent fractions in a deep way. This post will move students through the concrete (tools), representation (drawings), abstract (numbers and symbols) instructional sequence.

This is the time of year where I start to gear up for teaching equivalent fractions activities. The two main grade levels that have to tackle this foundational concept are third grade and fourth grade. Teaching equivalent fractions always feels daunting, but there are some fun ways that you can get your students working with them in a variety of settings. Using a bunch of different ways to teach it will ensure that you connect with every last one of your learners. After you’ve tried out different methods and activities, students will connect to their own best way. Equivalent fractions take a lot of time and practice, so it’s good to provide instruction in a variety of ways. The best part is the activities here can be used to teach equivalent fractions in both whole group settings and your small group teacher table, you just need to choose what works best for your classroom. Learning equivalent fractions in a fun way is also very motivating for kids! Some of these activities are fraction games, fraction number lines, and use manipulatives like fraction tiles, fraction circles and pattern blocks. The best part about teaching this way is that your fraction unit will be magical, and I want YOU to feel confident teaching fractions as well.

Start with the Foundation

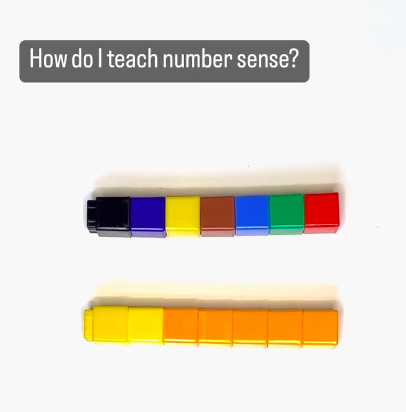
The first thing, and most important part of your fraction unit, is that students must have a feel for the size of fraction pieces. This usually begins in first and second grade when they work with halves and quarters, but some students seem to miss it. I like to call this fraction number sense, which is the building blocks or the general understanding that fractions are just special kinds of numbers that come in between whole numbers. They need to know what the size of particular unit fractions feels like. What I mean by that, is that with a little experience, they should automatically know that 1/10 is much much smaller in size than 1/3. If your students don’t know this yet (especially your older students in fourth grade), you must start there. You want to start with activities that help them understand the size of the pieces.

The first thing I like to use to establish this foundation is a fun game called Fraction Formula. Every student I play this with begs to play it again and again until I can barely stand it anymore. It is far and away one of my student’s favorite things.This is a great small group center once they are introduced to how to play the game. The object of the game is to take turns drawing a fraction card which all have different names, and get as close to 1 (1 is the top of the test tube) without going over. The nice thing about the game is that they get touch and physically handle the pieces (which coincidentally are benchmark fractions that they need to know!), and they see very quickly how it will pile up. They learn quickly which card they do NOT want to draw because the piece is too big. It is also a great activity because it naturally helps them with comparing fractions as well. I highly recommend this game as it goes beyond fraction circles and fraction tiles, giving them exposure with a manipulative that is highly engaging. I guarantee your whole class will love it.

Once you feel like your class has a good understanding of the size of the pieces of fractions, then it’s ok to move on to manipulatives to explore equivalence.

Exploring Different Types of Manipulatives (Concrete)

The next step in learning equivalent fractions is to explore different types of manipulatives using concrete models. Hands-on activities are really important for understanding fractions, because this leads to being able to use visual representations. Using visual representations is important for taking high stakes state tests-as they aren’t allowed to have concrete materials. Lots of classroom instruction is a great place to work in a hands-on way with these concepts so they don’t feel blindsided by the test.

Play-Dough First

The best way that I can think of to give real world experience with fractions is to use play dough. This is my all time big hitter great hands-on activity. Kids have so much fun using play-dough and they can screw it up as many times as they want and it’s easy to start over. However the most important part is to pretend that the play dough is different kinds of food items. Without the real world connection the point of the playdough is lost. The reason I love this, is that it lends to drawing fractions models easily, helping them be accurate with making equal parts. Here’s how you do it:

Have your students make several of the same size of “cookies”. The whole has to be the same size. I like to use a square shape because it’s easier to make, and easier to cut. We “cut” our cookies using popsicle sticks for safety purposes.

Tell them to cut different unit sizes, ensuring they are “cutting” the right sizes. Each piece after cut should be equal in size. As soon as they have made the cuts look equal, have them draw a visual model of it. Drawing the visual models is key to helping students move forward in their fraction thinking.

Using Playdough to Help with Visual Models

When you can see your students successful with square cookies, you can have them try to unitize other shapes (e.g. a candy bar rectangle, or a pizza circle). Half might look different with different original whole shapes!

Half is different with different sized wholes.

Fraction Tiles – Hide the Labels

If you’re a follower of my blog, you know that I love to flip over fraction bars so that the label is NOT showing. You’re probably thinking that’s nuts, and how are they supposed to know what the pieces are? Well, when it’s written on it and given to them they stop thinking. What we need them to do is reason about the size. Without seeing the labels, they will very quickly find out the size of each piece by counting how many of them fit in one whole. After they’ve got a handle on the size of the different fractions, then you can start asking them to find equivalence by comparing the pieces.

Fraction Circles without Labels

Fraction Circles are just like fraction strips or fraction bars, a great way to get students to figure out what size the pieces are. They work best when you use them without any labels. Same drill with these, show the whole number piece and have the students figure out the size of the pieces. Once they have named the pieces, have them try to find and record as many equivalent fractions as they can.

Pattern Blocks

Pattern blocks are another tool that many of us have, but typically only bring out for geometry. These also do not have labels, so in the same way you can use fraction strips without labels, you can have them explore the size of pattern block fractions.

After they have figured out the sizes, they can do the same thing that you did with fraction tiles, lay them on top of one another to find equivalent fractions. I like to have them write it out in words to help them make sense of the fact that they are still relating their fraction pieces to whole numbers.

Here’s a pro tip, have different groups with each of these materials, and have student record their findings on a giant anchor chart. Start to look for patterns!

Moving to Visual Models (Representation)

Once you feel like your students have a strong understanding of the hands on tools, then you will want to move to visual models for your equivalent fractions lesson. When students can draw visual models, they can actually visualize the tricky concept of equivalence. For example, if they draw a square and cut it in half, they can shade in one half of the square. One more cut doubles the size of the pieces, changing the size of the pieces (denominator) to fourths and changing the number of pieces shaded in to two.

This quick and simple visualization explains why you can multiply the numerator and denominator by the same number. All you need is a piece of paper and a drawing utensil. This is not an equivalent fractions worksheet, rather it’s a way for you to help students generate their own equivalent fractions. Worksheets often have the visuals drawn for them, and that takes away much of the learning. When you teach this way, you don’t have to have students remembering rules or tricks about when they have the same denominator or same numerator!

You can also use a fraction number line to help solidify this concept. Number lines can seem so abstract, but they can be less so when connected to something real life.

Please read about my driving example here and give it a try.

Applying the Knowledge to Real Life and Testing Situations (Abstract)

If, and only if you feel that students have a solid understanding of these concepts, can you finally introduce the idea that equivalent fractions can be found by multiplying or dividing the numerator by the same number. This is the shortcut that most of us were taught when we went to school, but we had nothing to connect it to. See this quick instagram clip to see what I mean:

Of course you know that I love real world connections, and I especially love when the real world connections turn into anchor charts. Have your students share equivalent fraction examples that they can find in real life! A little time spent on this important concept will go a very long way in their understanding of equal fractions.

For example:

2 quarters of a football game are in one half of the entire game! That amount of time is the same.

If you are cooking, and you can’t find your quarter cup, you would need two eighth cups to have the same amount of sugar.

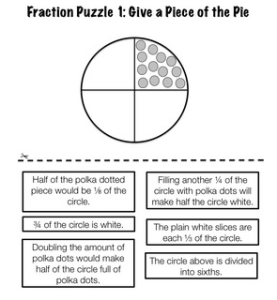
Another way I like to have them apply their knowledge is to do sorting activities. These kind of fraction lessons are great activities for really digging deep into this concept and applying what is known to “test like” questions. I cannot stand the thought of sending my students unprepared for state testing. Providing rigorous activities is an easy way to use the time at your teacher table to try to squash misconceptions. The photos below show how you can use these two different activities to determine which fraction concepts might still be shaky. They include answer keys as well so that you can quickly see if they are understanding.

In Reasoning Puzzles, students decide if statements are true or false based on the visual given.

In Piles, students turn over a fraction card and try to match it to an anchored card (the cards with the star) at the top. This is an awesome way for them to deeply explore equivalent fractions.

I hope this post has been a comprehensive way for you to explore some ideas for teaching equivalent fractions. I’d love to hear more about what you do in the comments!

January 30, 2023 by Beyond Traditional Math

5 Tips for Making Anchor Charts Like a Pro

It breaks my heart when I hear elementary teachers shamefully tell me that they were “never good at math”, hated math or just don’t feel confident. The truth is, if this is you, it is a GIFT. If you never felt confident in math, then you know exactly how your students feel. Not only that, it will make you an anchor chart machine.

Anchor charts are the missing link between our instruction and students practicing mathematics. We as teachers may have taught a concept 5 years in a row or more, but for students it could be their first time hearing it. They also may have holes missing in previous years instruction for a variety of reasons. Giving students what they need right up on their wall is the first step in helping them make connections, remember complicated processes or learn brand new vocabulary. You have English Language Learning students? Special Education students? It doesn’t matter who is in your classroom, anchor charts are good for ALL of your students.

Take this anchor chart for a grade 2 unit on number lines for example:

There are things on that anchor chart that every single adult takes for granted. We know what a tick mark is, we know what a point is, and we know what to do when numbers are missing. These are things that the majority of our students have never experienced. This anchor chart is especially important because number lines come up in so many concepts for many years to come.

Anchor charts are best made when you think about the following 5 things:

How can we make it together? This is critical. When learning is synthesized at the end of a lesson, that’s the perfect time to add to your anchor chart, and as much as possible written in student friendly language.
What new math specific vocabulary needs to be explained on the chart? Act like a new learner, like you’ve never heard some of these words before. Which belong on the anchor chart? How can words be written with precision, but still student friendly? Anchor charts explain that we can use math words like point instead of dot or tick mark instead of line.
What misconceptions do students have? What is important to remember, and what errors are you are seeing your students make in the lessons?
What is the least amount of words I can put on it? Visuals are KEY. The more visuals you have the better! They are both engaging and beautiful. Some of the anchor charts I’ve seen are absolute works of art.
How new is this material to my students? If they haven’t had prior exposure to the concept, it MOST definitely belongs on your wall.

This is not an exhaustive list, but a place to start. The more you work on anchor charts the better you will get at them. As you walk around helping, you’ll notice a lot of the same questions keep on getting asked. This is an instant signal that you need to get that up on the wall to help mass amounts of students.

I wish this is something that I would have known when I was a first year teacher, when I thought I was bad at teaching math. It would have been an incredible thing to really think through all those things that I struggled with as a student, learn along side them and provide the proper supports.

January 28, 2023 by Beyond Traditional Math

3 Tips to Help Students Tell the Difference Between Area and Perimeter

For as long as I can remember students have mixed up the difference between area and perimeter on standardized tests. Around this time of year as state testing approaches, we all get this nutty feeling that we need to start hammering test prep activities and get out flashcards with vocabulary. Here are three easy ways to ease your test prep anxiety and get students engaged with these two concepts.

First: make it part of your every day practice. For example, when it’s time for a class meeting and you’d like them to sit in a circle, make it a rectangle instead and ask them to sit on the perimeter of the mat/rug. “Please go to your perimeter spot.” When it’s time for a mini lesson, have them sit in the area part of the mat. “Please have a seat in your area spot.” Take a picture of them in both of those spots and label it on an anchor chart during your beginning of the year routines.

Second: use visuals like anchor charts during your lesson, and then keep up after your lesson on your walls. Check out this real world connection from Fifth in the Middle:

Once they understand the real world application, you can then talk through a more abstract model for your anchor chart (which is much more like what they will see on a standardized test):

Third: Try some deep thinking activities that will get them applying the concepts. Press play on the video below to see it in action. You can try one for free by clicking the link for PILES and downloading the preview. The activity is SO engaging and sometimes more effective because it’s not a worksheet. I love it because we have so many representations on standardized tests, and this takes some of the pressure off of test prep.

January 25, 2023January 25, 2023 by Beyond Traditional Math

Teen Numbers are Early Place Value

Teen numbers in English are STRANGE. I mean, look at these weirdos in the first half of the numbers:

11 – eleven (not one teen)

12 – twelve (not two teen)

13 – thirteen (not three teen)

14 – fourteen

15– fifteen (not five teen)

16 – sixteen

17 – seventeen

18 – eighteen

19 – nineteen

When we start teaching these numbers to our youngest learners, we often take for granted the strangeness of the names of these numbers.We are used to the names and counting with them…we don’t even question or notice how strange they are. The first half of them don’t even follow a logical pattern! In addition, I often hear videos being played where the singer is saying “Teen numbers start with a 1!” Actually, teen numbers are written with a 1 first yes, but that one in the teen number does NOT have a value of “one”.

Teen numbers are the basis of place value. I still work with third graders who will see 10+6 and start counting on their fingers to figure it out! How they don’t know that shortcut always shocks me. I think somewhere we failed them in understanding the value of the 1 in the teen number.

Beginning to learn about place value for our early learners needs to include some key things. We have to spend a lot of time letting them play with the numbers, get comfortable with different representations of those numbers, including finding the ten that is hiding in the number. In many state standards teen numbers are expected to be thought of/written as a group of 10 and some more ones. YES, this is key! In the photo below you’ll see some of these various representations with fingers and numbers. In future posts, I’ll show some other representations and tools that work AWESOME.

In addition concrete tools should also be incorporated as much as possible! Here we are using units of one and ten to make it match the fingers. Very powerful for them to connect a tool from their body (fingers) to a more abstract tool (base ten blocks).

Never skimp on those teen numbers kindergarten teachers! Future grade levels depend on you to explore these concepts deeply with your students.

January 19, 2023 by Beyond Traditional Math

How to Subtract and Regroup Mixed Numbers with Visual Models

Flashback to 4th grade when my math teacher asked me to subtract mixed numbers, my palms were sweaty, and I felt like I was looking at the demonstration cross eyed WITH brain fog. If you are a loyal follower of my blog, you might know about my past traumatic experience with fractions. It was not pretty, and the last thing I want is for any student of mine to feel this way.

I created the video below to try to show regrouping with visual models. I can’t stress enough how visual models have changed and revolutionized my teaching. It almost never fails that when students struggle, I’ve forgotten to include a visual to help them “see” the math. I am not alone, check out Dr. Jo Boaler, Berkeley Everett and many others who truly understand how we can deeply teach mathematics through visual representations.

The video below will speak for itself, but if you don’t have time to watch it (it’s less than five minutes), the idea is that you can regroup whole numbers into fractions just like students do with base ten models. It’s SO MUCH FUN when students get to learn this way with you. Please check it out and let me know what you think!

January 14, 2023January 20, 2023 by Beyond Traditional Math

Start Geometry Vocabulary Early!

I love to be sneaky with using vocabulary in the classroom. One of the ways that I like to embed it is through game playing. For example, instead of using two color counters, we pull out the pattern blocks. Instead of saying “I want blue!”, I tell the students that I would like them to sound more like a fancy mathematician. Instead you must say, “I would like to play with the trapezoid, please.” It causes a laugh with the kids, but even better it starts to get them to use those words more regularly and with purpose. Plus they love to say the word trapezoid! There is a nice benefit to getting these words in their mind early, they’ll start to connect that vocabulary to real world examples of lines, points, segments and other geometry terms.

There is a natural reason to determine the difference between the two tools in the photo below, and to examine how the rhombus and trapezoid are different so that they know which one to pick. This can start as early as the primary levels! Most pattern block sets come with hexagons, triangles, rhombuses, parallelograms, and trapezoids.

January 10, 2023 by Beyond Traditional Math

Math Facts and Fingers

Should we allow students to use their fingers when learning and solving math facts? I’m going with an emphatic YES…with one condition. They have to be using them efficiently. For example, are they solving 10 – 4 by putting up one finger at a time to show ten fingers, and then removing one at a time? That might not be the best finger usage. However, all 10 fingers up and putting four down in one “poof” makes TOTAL sense mathematically. We are letting students make sense of quantity and structure numbers when we do this.

Check out my instagram post to see this in action by clicking the photo above.

There is abundant research out there about finger use, and the deep connections it makes for our student’s fact fluency knowledge.

The best part? Students feel SO confident with learning subtraction facts, which is usually much harder to teach and learn than addition.

January 8, 2023 by Beyond Traditional Math

I’m Taking a Big Risk!

I used to hate teaching math when I began my education career. It was scary! I didn’t know what I was doing and had ZERO confidence. 18 years later, you’ll find me traveling across the country teaching other teachers the best ways to teach math. My number one goal in starting this blog is to help YOU love to teach math. However, blogging isn’t the main form of learning these days. I find myself going to social media to learn so many things. So…I am (very nervously) trying a new idea where I do things way beyond my skill and comfort level:

I have started an instagram account (a professional one), you’ll find it by clicking here: @beyondtmath
My goal is to release as many teaching tips as I can using reels or video posts.
I want every video to be under 60 seconds, and something you can try immediately.
I plan to try to do these weekly.

It feels worrisome to put myself out there, but I’m going to be posting all those tips that I tell teachers and I hear back: “I wish someone had told me this!” or “I love that idea!” or “I am SO trying that!” or “That finally makes sense to me!”

My first post is about how to develop number sense with cubes. I hope you’ll venture over and find me! (Please, please, please!)

January 7, 2023 by Beyond Traditional Math

The Absolute BEST Way to Practice Multiplication Facts

Flashcards are the worst for practicing fact fluency. I’m just going to say it. They are JUST the worst. Actually maybe timed tests are the worst, but we will tackle that another day. Talk about taking all the joy and fun out of mathematics. They only work for students who can memorize, and while you might be thinking “Hey! They worked great for me!” You were probably one of the lucky ones. Even if they DO work for SOME kids, is that the best way to practice math facts?

My favorite, favorite, favorite thing is to have students play with multiplication first. Multiplication fact practice doesn’t have to be devoid of all fun. You start out by purchasing really great things that are high interest for children, and then find a way to help them put them into groups. We use fake eggs and egg cartons, pop its to show rows with pressed in dots, penguins on icebergs and the fan favorite among every student I’ve ever done this with…is the mini shopping carts with groceries.

Practice multiplication facts with fake eggs

As long as the items can be put into groups, they can represent multiplication. Now in this case you can actually pair flashcards with these items. Pick a flashcard, then build it. This will help your students make some meaning out of the number sentences. The more students build their multiplication facts, the faster they learn the meaning of multiplication conceptually. The faster they learn the conceptual part, the easier time they have visualizing multiplication. The easier time they can visualize multiplication facts, the faster they can learn strategies for skip counting or doubling groups. It snowballs quite quickly! I’m a huge proponent of games and activities like this vs. time tests and flashcards. This does a lot of great things for students:

It reduces anxiety big time and adds in play, which research shows allow you to be a better problem solver.
Students start to look for shortcuts (our brains are wired this way), and they will begin to skip count rather than count by ones to find the total.
It normalizes the use of tools for ALL students.
Eventually these items won’t be needed, as they will eventually become tired of building the items and will come up with other ways to solve multiplication facts.

This is an awesome thing to work on at your teacher table, or to have as an ongoing station for students to play around with during independent work time in your math workshop.

January 4, 2023 by Beyond Traditional Math

Real Life Examples of Multiplication

The switch from working with addition/subtraction to multiplication/division for students can be pretty jarring. With multiplication, everything they have previously known about abstract symbols suddenly changes from straight up just adding what you see to adding groups or rows. While many students can successfully use multiplication, I will often see the writing of number sentences to be full of small errors. I’ve found the best way to clear up those errors is to use really engaging REAL WORLD multiplication examples.

For instance, in the image below, you can see five lily pads with 3 frogs on each lily pad. The number sentence that matches this story could be 5 x 3, because there are 5 groups of 3 frogs. I love to help them make sense of the symbols and numbers by simply labeling that number sentence (see pic below), and then having them work through several examples.

You can have them practice this skill in a few ways:

Write the number sentence and have them build it. Do it over and over, until they get used to the first number being how many group they need.
Build it for them and have them write a matching number sentence.
Give them a number sentence, build it incorrectly and then have them find your error. (THEY LOVE THIS!)

The more practice they get with these concrete items, the faster it will settle in, and the more confident your student will feel!