November 11, 2014November 11, 2014 by Beyond Traditional Math

What Exactly IS Subitizing?

For a few years, I saw the word “subitizing” and I had no idea what it meant. It is one of those things where you don’t know the word very well, but once it enters your consciousness, you start to see it more and more.

Last summer I learned what subitizing is (I pronounce it soob-i-tizing, some people say sub-i-tizing), and how important it is for K-2 students to be able to do. Quite simply, subitizing is the ability to count a collection of items without having to count each item individually. For example, when you roll a dice you automatically know the number without having to count each dot individually.

Subitizing is important because it means that children can hold an image in their minds. They are able to group collections effectively so that they no longer need to count by ones. We want them to do this with regular patterns and irregular patterns. You know that children are subitizing if they can tell you the number in the collection when it is flashed at them quickly.

Here is an example of an irregular pattern, and what we want the student to think in their minds:

We want students to group items together to count efficiently.

There are several videos (if you google it) that people have created to help children practice this skill. Here is just one (warning: it flashes fast!):

How do you have students practice this skill in the classroom?

February 27, 2014 by Beyond Traditional Math

When Number Sense is Missing: Extend Patterns

Sometimes math is like a stinking onion. You encounter a student who is having a problem, so you peel back a layer to discover more misconceptions which leads to more and more problems until you have a big stinking mess and lots of tears. The good thing though, is a stinking onion can be cooked in a way that tastes delicious.

We are deep into our division unit, and many students are struggling with strategies to divide by 8 and 9. These are much larger numbers that we are working with, so the most fragile students are even more sensitive as they calculate their answers. Red faces, frustrated brows, and a lack of perseverance sets in quickly.

A student that was trying to divide 48 by 8 was really struggling. She had already tried to solve it by making equal groups, she had tried repeated subtraction, she had tried to figure out the related multiplication fact, but she kept getting lost in the process. We decided to find the related multiplication fact together.

I asked her to try thinking of a friendly eight fact. 8 x 2 felt good, she was able to solve that. She then jumped to 8 x 5. She counted by fives until she had 40. I waited to see if she’d make the connection that 8 x 6 is just one more eight added to the group. I waited, and waited. Nothing. So I wrote it out for her…8 x 6. I asked her, “What is 40 and 8 more?”

Nothing.

I mean, there was complete and utter silence.

I was actually speechless that she made it this far and didn’t know how to add 40 and 8! In a calm and non-judgmental way, I wrote out the following sequence on a dry erase board:

0 + 8

10 + 8

20 + 8

30 + 8

40 + 8

After she solved the first two, she immediately saw the pattern and we both breathed a sigh of relief. I didn’t leave the school day feeling very good though. This concern has been in the front of my mind all night. How is this child supposed to learn to multiply and divide when her number sense is so fragmented?

So many of us (I am guilty of this myself) have stressed memorization of math facts without any strategy or number sense behind it. This moment today has convinced me that this type of practice MUST happen regularly for students, and those strategies need to be shared out loud often. Extending patterns is essential for students to become strong mathematicians. It is part of the math practice standards and truly is an important skill.

How do you promote number sense (or extend patterns) in your classroom?

February 23, 2014 by Beyond Traditional Math

“Aren’t You Teaching Them To Find the Wrong Answer?”

“I just don’t understand estimation. Aren’t you teaching them to find the wrong answer? Why don’t you just teach them to find the exact answer to the problem?”

These were the words that a parent said to one of my colleagues in my building at her parent teacher conference. The parent wasn’t angry, but was firmly questioning. My colleague told me that she was very caught off guard, because the new math series we are using is very “new” to her as well in her thinking. She is a veteran teacher of 22 years, and was taught to carefully calculate when she learned math. She was taught that there was ONE right answer, and that math is about finding that one answer. She has had trouble being fully on board with estimation as well. She came to me because she was looking for what to tell this parent, as it wasn’t the first time she had heard this comment.

Whenever I’ve had this question, I usually present a scenario like this to parents:

Without estimating (either before or after solving), many students make errors without even realizing it. Teaching them to estimate allows them to practice the Standards for Mathematical Practice, especially standard number 1. This standard expects students to make sense of problems and determine if their answer is reasonable. It is about thinking DEEPER, not just quickly or blindly solving problems without knowing if you are on track. Estimation can help us find errors in procedures like the one above.

Teaching children to estimate does not teach them to get the wrong answers, it teaches them to think. Practice it the right way and students will become stronger mathematicians.

January 11, 2014January 26, 2023 by Beyond Traditional Math

Give the Dog a Bone: FREE Number Sense Game Online

Give the Dog a Bone is a great little game for early learners (or for those students who struggle with number sense), encouraging them to think about patterns to find numbers on a blank number line. In the game you need to find 10 numbers on a blank 100 chart in 1 minute. It is pretty fun and addicting!

What I like best is that the added time pressure encourages them to think about patterning, rather than simply counting one square at a time. For example, in the situation below they might count by tens to get to sixty one, rather than count 61 squares. To get all ten bones in a minute, they’ll need to think this way!

This game could be used as a link from school to home, as an activity in the computer lab or with small groups!

January 1, 2014 by Beyond Traditional Math

Even and Odd: The Importance of Conceptual Understanding

I can’t tell you how many times I learn through the eyes of my five year old. She asks amazing questions, especially when it comes to numbers. While we were doing some daily math play today, I thought about introducing her to the concept of even and odd numbers. I was taught even and odd by being told to memorize the numbers: 2,4,6,8. As a tiny child I don’t think I truly understood why a number was even vs. odd.

So we built the numbers 1-10 (in hindsight I wish I had included zero) with unifix cubes. Then I told her to put them in pairs. We put the pairs together, and lined everything up. During this process she was uncomfortable whenever one was left over, and wanted to pair it up with one from another number set! This is how it looked once we had it all situated.

Then I asked her what she noticed.

“There is ‘ones’ all by itself!”

We pointed out which numbers had an “odd one out” as I put it. That was when I introduced the words odd and even. I think it is essential to introduce the vocabulary to kids after they have explored the concept, so that they have a way to name what it is they are seeing.

She then noticed that every other number was odd. So we looked at and extended the pattern: odd, even, odd, even….until we got to the 10. I then asked her what she thought 11 would be, odd or even? She shouted out “odd!” without even thinking about it.

I still have a few third graders that are struggling with this concept (can you even believe that?). I am going to try to see if this could help them understand this very basic concept.

December 31, 2013December 31, 2013 by Beyond Traditional Math

Math Play: 20 Minutes a Day

I really think it is amazing that as a society we have really been pushing new parents to read to their children for 20 minutes a day. We all know that this helps a child in a myriad of ways to become better speakers, writers and readers. I am blessed with a five year old that learned to read completely on her own at age 4, and I believe that reading to her every day of her life has been the reason this happened. The cool thing was, we didn’t have to teach her to read, it just happened on it’s own (trust me, we were shocked and humbled by this development!). Whether that is the norm or not, it got me thinking about math.

Why don’t we as a society promote 20 minutes of math play each day, the same way we promote reading? Imagine if children could authentically develop number sense from an early age by counting or playing with numbers DAILY. I bet we wouldn’t see as many students struggling with some of the simplest concepts like even vs. odd. I still have a few third graders who aren’t sure of the difference!

This is my daughter “building” numbers today. All we needed was a little paper and some unifix cubes which you can pick up at any educator supply store. I want her to see that numbers aren’t really all that abstract. We’ll be adding many different number representations to our papers each day.

I plan to add math play to my 5 year old’s day, the same way we read each day. If you already do something like this, I hope you can share some of your activities with me!

Last, this also gets me thinking about my third graders. Is there a way that I can have math play be homework? Hmmm….I’m on it!

November 17, 2013December 30, 2013 by Beyond Traditional Math

Tricks are NOT for Kids

Rounding is one of the worst things in the world to teach, right? It is classic every time. Mention rounding…kids sigh, teachers tense up and everyone’s brains feel muddled. It is HARD to understand rounding when you don’t have strong number sense.

I used to teach every trick under the sun for rounding. I tried the rounding mountain, I tried the rounding coaster. I even would break it down for kids and have them underline the place, circle the number next to it, check to see if it was 5 or higher. There were SO MANY problems. Kids couldn’t figure out the place value, where to start, what to change, what to look for or even what the number was in the end. A few students would understand it, and it would stay that way.

That was when I realized they weren’t understanding the concept behind the tricks. They couldn’t remember the rules of the tricks (not even when it was a rhyme, because they only memorized the rhyme and didn’t get how to use the trick).

So I threw all of it away. I took down all of my rounding roller coaster and rounding mountain anchor charts. I decided to start fresh. I told my students this year to FORGET everything they ever learned about rounding.

Then, we counted by tens. Not just 10, 20, 30, 40, 50 60, but also 110, 120, 130, 140, 150. We counted by hundreds in the same way. We talked about what nearest ten and hundred mean. We made number lines that counted by tens and hundreds. THEN, we began rounding numbers.

And this is what we came up with as a class:

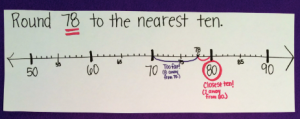
It was so simple. If you are asked to round to the nearest ten, begin counting by tens by making a number line. Find the closer ten by checking how many spaces away it is from your number. As we worked with more numbers, they uncovered the mystery of the 5. “What do we do if it is in the middle?!” We round to the higher ten, because that is what the world decided to do so that everyone does it the same.

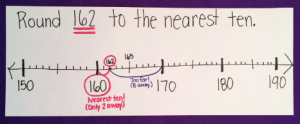
We could also round to the nearest ten in numbers in the hundreds, because we practiced counting by tens in the hundreds.

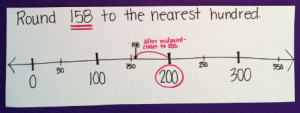
We can also round to the nearest hundred in this same simple way!

This whole entire thing was AMAZING. After several days of practice students weren’t drawing out number lines anymore, they were able to picture them in their heads.

On my pre-test I had 5 students who could round to the nearest ten and hundred. By the post-test every student except for ONE student could do it. Only one! I have been able to sit down with that student each day as he comes into school, and he’s getting it now, too.

It made me realize that tricks really aren’t for kids. When students don’t understand the WHY behind the trick, the tricks don’t work. If they don’t understand the number sense behind rounding, no matter what number they underline or look at, it won’t be solid conceptual understanding, and the trick will get mixed up.

Beyond Traditional Math

Fostering conceptual understanding with real world connections.

Tag / number sense